How to Solve Equations with Fractions

Solving Equations with Fractions Using Addition

In our first example, our equation is b - 11/10 = -13/5

We need to find out what the variable, 'b', is. A variable is a symbol that stands for an unknown number. To do so, we have to isolate the variable, meaning that we have to have the variable on one side of the equal sign by itself. First, we add -11/10 + 11/10, which equals 0. Now remember, whatever you do on one side of the equal sign has to also be done on the other side of the equal sign. So we add -13/5 + 11/10, which is -15/10. The current equation is now b = -15/10. We have finally found out what 'b' is equal to! But, we have to simplify -15/10, which is -1 1/2. Now the correct answer is b = -1 1/2

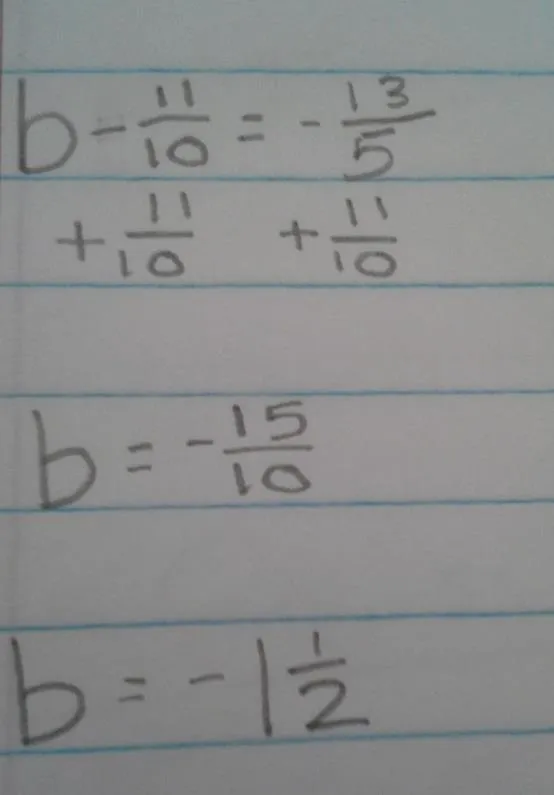
Solving Equations with Fractions Using Subtraction

In our second example, our equation is 5/6 = x + 2/3

Like we did in the first example, we need to isolate the variable. Subtract 2/3 - 2/3, which results in 0. Then, also subtract 5/6 - 2/3, equalling 1/6. The equation is now 1/6 = x. Since 1/6 can't be simplified, 1/6 = x is the final answer.

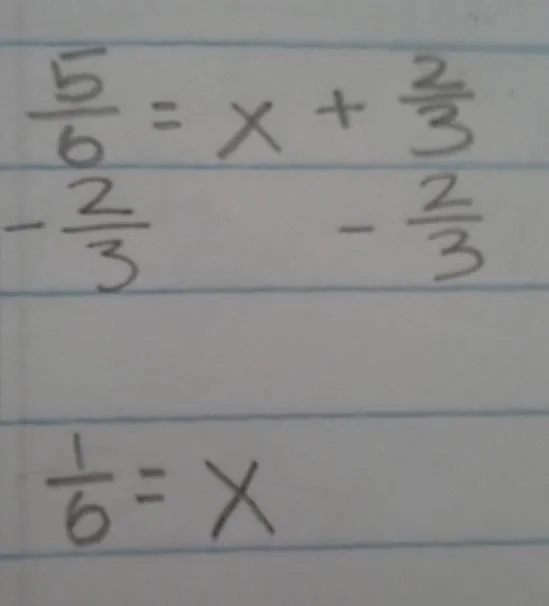
Solving Equations with Fractions Using Division

In our final example, our equation is 3 1/4j = 3/2

Before we do anything at all, to make it easier on ourselves, we must convert all mixed numbers into improper fractions. The only number we need to convert is 3 1/4 and as a improper fraction, it is 13/4. The current equation is now 13/4j = 3/2. Again, we need to isolate the variable so we divided 13/4 by 13/4. On the other side of the equal sign, we do the same thing by doing 13/4 divided by 3/2, which is 6/13. We finally found out that j = 6/13 and that this is the final answer since 6/13 can't be simplified.

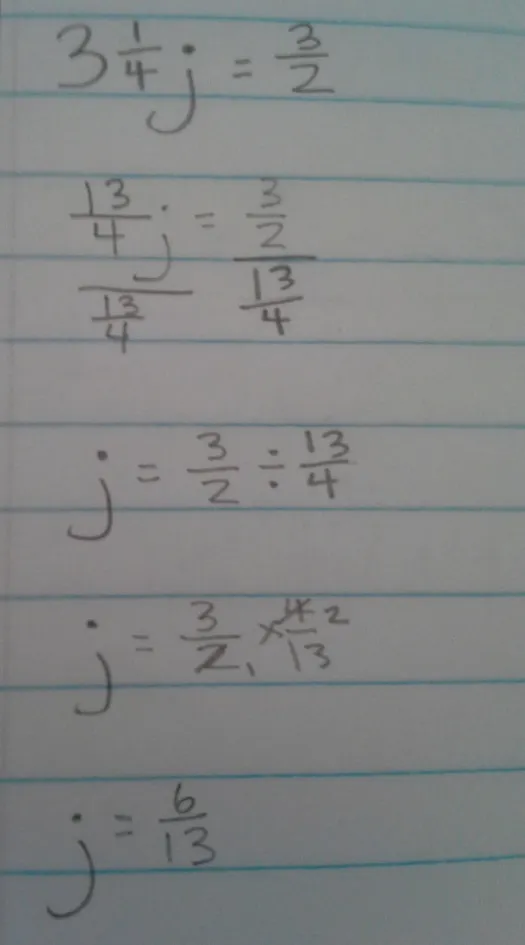
Practice Time

Solve the equations below

1. a - 3/5 = -7/5

2. v + 3 4/9 = -46/9

3. 15/2 = 3/5x

Answers:

1. a = -4/5

2. v = -77/9 = -8 5/9

3. x = 25/2 = 12 1/2